
electrons interacting with the lattice of a metal) tends to be somewhat heuristic or even a bit arbitrary.Īnother approach uses partial molar quantities. Furthermore, parsing of the entropy between interacting components of a system (e.g. An important property of this approach is that it is entirely model-dependent. Beyond this there is a hierarchy of increasingly sophisticated models of increasing realism, usually based on concepts from the band theory of solids.
#Molar entropy free#
The simplest model is the Sommerfeld model, which is based on the properties of a degenerate free electron gas. One takes a microscopic view, based on models of electrons in metals and superconductor and uses statistical mechanics to calculate the total thermodynamic functions for the model-based system. There are at least two valid approaches to the thermodynamics of electrons in metals.
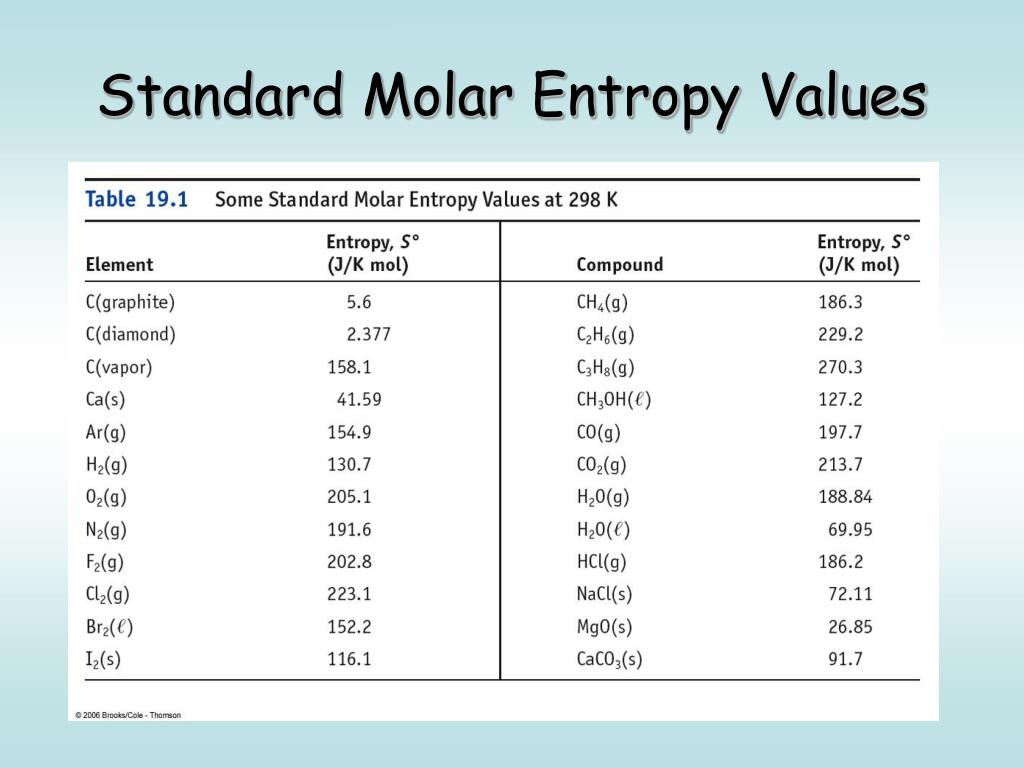
Received 9 November 2015 accepted 26 January 2016 published 29 January 2016Įntropy and heat capacity measurements have long played an important role in providing insight into the electronic structure of metals and superconductors. A unifying principle between reversible and irreversible thermodynamics is also discussed, including how this relates to the completeness of thermodynamic theory. Furthermore, the application of partial molar quantities to electrons in metals and superconductors has direct relevance to long-standing problems in other fields, such as the thermodynamics of ions in solution and the thermodynamics of biological energy transformations. This provides information that is complementary information from other approaches to the thermodynamics of electrons in metals and superconductors and can provide additional insight into the properties of those materials. The present paper will explore the application of partial molar electronic entropy and partial molar electronic heat capacity to electrons in metals and superconductors. Partial molar quantities have not been widely used in the field of solid state physics. Another uses partial molar quantities, which is a rigorous thermodynamic method to analyze systems with components that can cross phase boundaries and is particularly useful when applied to a system composed of interacting components.



 0 kommentar(er)
0 kommentar(er)
